olzcolors.blogg.se - Is there a font that looks like a 7 segment display

Eight letter display, but custom expandability.

Here are the features of our 7-segment scrolling text display: In this article we will go through all necessary steps so that you can easily build your own! Let's go :) time ago we learned how to use shift registers with microcontrollers, and in this project we will use them together with the PIC16F1455 microcontroller to build a scrolling text display. Let's implement the above approach in a Java program. So, the total number of segments that we require is 28 (5 + 5 + 4 + 5 + 6 + 3). The minimum number of segments requires to represent digit 7 is 3. The minimum number of segments requires to represent digit 6 is 6. The minimum number of segments requires to represent digit 5 is 5. The minimum number of segments requires to represent digit 4 is 4. The minimum number of segments requires to represent digit 3 is 5. The minimum number of segments requires to represent digit 2 is 5. Suppose, N=6 (number of digits) and s="234567". Sum up all the segments and return the total number of segments to display the N-digit number.

We have given an N-digit number and we have to find the minimum number of segments required to display each digit. Let's discuss the subproblems one by one. In the second subproblem, we will find the element that uses the minimum number of segments to display a number.In the first subproblem, we will compute the minimum number of segments required to display a number.

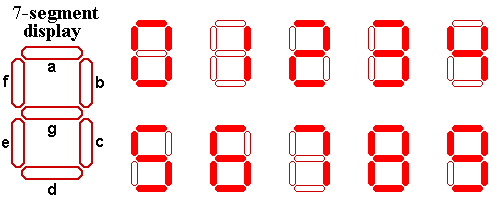
The problem is divided into the following two subproblems. Return ntainsKey(digit) ? encodings.get(digit) : 0x00 Private static void lightSegment(boolean on, String onValue, String offValue) the function decides which segment to be on or off print method that computes how many segments are required to print a digitĬhar bits = String.format("%7s", Integer.toBinaryString(code)).replace(' ', '0').toCharArray() calling the print method that prints the digit on the console loop runs from 0 to 9 and print digits one by one by invoking the print method key-digit, value-hexadecimal encoding for displaying the digits 0 to 9 (in abcdefg sequence) static block that stores key and corresponding values to the Map Private static final Map encodings = new HashMap() Let's implement the above logic in a Java program. In the table on represent, 1 and off represents 0. In the following Java program, we have used hexadecimal encoding to represent a digit in seven segment form. Let's see another way to print digits in seven segment display. Public static void main(String args) throws Exception